05th Apr 2019 @ 11 min read
- Examples Of Avogadro's Law
- Avogadro's Law Formula
- Avogadro's Law Equation
- Avogadro's Law Real Life Example
Avogadro showed us the sheer number of atoms that make up the universe. Avogadro’s number, which is 6.022 times ten to the 23rd power, are the number of atoms in a single mole of any element. In hydrogen that would equal one gram. “So what?” you m. Amadeo Avogadro was an Italian physicist who stated, in 1811, that the volume of any gas is proportional to the number of molecules of gas (measured in Moles – symbol mol). In other words if the amount of gas increases, then so does its volume. Avogadro’s Law in action (©2020 Let’s Talk Science). This blog is created as part of Faith Committee’s Performance Task in Science 10. It is all about Amedeo Avogadro’s Gas Law; what it is, and some real life examples of it. We aim to explain the assigned gas law to the readers. To navigate, please use the sidebar found on.
Avogadro's law is also known as Avogadro's hypothesis or Avogadro's principle. The law dictates the relationship between the volume of a gas to the number of molecules the gas possesses. This law like Boyle's law, Charles's law, and Gay-Lussac's law is a specific case of the ideal gas law. This law is named after Italian scientist Amedeo Avogadro. He formulated this relationship in 1811. After conducting the experiments, Avogadro hypothesized that the equal volumes of gas contain the equal number of particles. The hypothesis also reconciled Dalton atomic theory. In 1814 French Physicist Andre-Marie Ampere published similar results. Hence, the law is also known as Avogadro-Ampere hypothesis.
Statement
For an ideal gas, equal volumes of the gas contain the equal number of molecules (or moles) at a constant temperature and pressure.
In other words, for an ideal gas, the volume is directly proportional to its amount (moles) at a constant temperature and pressure.
Explanation
As the law states: volume and the amount of gas (moles) are directly proportional to each other at constant volume and pressure. The statement can mathematically express as:
Replacing the proportionality,
where k is a constant of proportionality.
The above expression can be rearranged as:
The above expression is valid for constant pressure and temperature. From Avogadro's law, with an increase in the volume of a gas, the number of moles of the gas also increases and as the volume decreases, the number of moles also decreases.
If V1, V2 and n1, n2 are the volumes and moles of a gas at condition 1 and condition 2 at constant temperature and pressure, then using Avogadro's law we can formulate the equation below.
Let the volume V2 at condition 2 be twice the volume V1 at condition 1.
Therefore, with doubling the volume, the number of moles also gets double.
The formation of water from hydrogen and oxygen is as follows:
$underset{1,text{mol}}{ce{H2O}}$}' alt='Water reaction'>In the above reaction, 1 mol, (nH2) of hydrogen gas reacts with a 1⁄2 mol (nO2) of oxygen gas to form 1 mol (nH2O) of water vapour. The consumption of hydrogen is twice the consumption of oxygen which is expressed below as:
Let say, 1 mol of hydrogen occupies volume VH2, a 1⁄2 mol of oxygen occupies VO2 and similarly for 1 mol of water vapour, volume VH2O. As we know from Avogadro's law, equal volumes contain equal moles. Hence, the relationship between the volumes is the same as among the moles as follows:
Avogadro's law along with Boyles' law, Charles's law and Gay-Lussac's forms ideal gas law.
Graphical Representation
The graphical representation of Avogadro's law is shown below.
The above graph is plotted at constant temperature and pressure. As we can observe from the graph that the volume and mole have a linear relationship with the line of a positive slope passing through the origin.
As shown in the above figure, the line is parallel to the x-axis. It means that the value of volume by mole is constant and is not influenced by any change in mole (or volume).
Both the above graphs are plotted at a constant temperature and pressure.
Avogadro's constant
The Avogadro's constant is a constant named after Avogadro, but Avogadro did not discover it. The Avogadro's constant is a very useful number; the number defines the number of particles constitutes in any material. It is denoted by NA and has dimension mol−1. Its approximate value is given below.
Molar Volume
Since Avogadro's law deals with the volume and moles of a gas, it is necessary to discuss the concept of molar volume. The molar volume as from the name itself is defined as volume per mole. It is denoted as Vm and having a unit of volume divided by a unit of mole (e.g. dm3 mol−1, m3 kmol−1, cm3 mol−1 etc). From the ideal gas law, at STP (T = 273.15 K, P = 101 325 Pa) the molar volume is calculated as:
Limitation of Avogadro's law
The limitation are as follows:
- The law works perfectly only for ideal gases.
- The law is approximate for real gases at low pressure and/or high temperature.
- At low temperature and/or high pressure, the ratio of volume to mole is slightly more for real gases compare to ideal gases. This is because of the expansion of real gases due to intermolecular repulsion forces at high pressure.
- Lighter gas molecules like hydrogen, helium etc., obey Avogadro's law better in comparison to heavy molecules.
Real World Applications of Avogadro's Law
Avogadro's principle is easily observed in everyday life. Below are some of the mentioned.
Balloons
When you blow up a balloon, you are literally forcing the air from your mouth to inside the balloon. In other words, you are filling more moles of air in the balloon and it expands.
Tyres
Have you ever filled deflated tyres? If yes, then you are nothing but following Avogadro's law. When you pump air inside the deflated tyres at a gas station, the amount (moles) of gas inside the tyres is increased which increases the volume and the tyres are inflated.
Human lungs
When we inhale, air flows inside our lungs and they expand while when we exhale, the air flow from the lungs to surroundings and the lungs shrink.
Laboratory Experiment to prove Avogadro's law
Objective
To verify Avogadro's law by estimating the amount (moles) of different gases at a fixed volume, temperature and pressure.
Apparatus
The apparatus requires for this experiment is shown in the above diagram. It consists of a U-tube manometer (in the diagram closed-end manometer is used, but opened-end manometer can also be used) as depicted in the figure, mercury, a bulb, a vacuum pump, four to five cylinders of different gases and a thermometer. Connect the all apparatuses as shown in the figure.

Nomenclature
- V0 is the volume of the bulb, which is known (or determined) before the experiment.
- T is the temperature at which the experiment is performed, which can be determined from the thermometer (for simplicity take it as room temperature).
- P is the pressure at which the experiment is performed, which can be determined from the difference in heights of mercury level in the manometer.
- W0 is the empty weight of the bulb, and it is known (or determined) before the experiment.
- W is the filled weight of the bulb.
- Wg is the weight of the gas inside the bulb.
- M is the molar mass of the gas.
Procedures
- Take a gas cylinder attached it the bulb setup and also attached the pump to the bulb setup. Care must be taken while attaching the apparatus to prevent any leakages of the gas.
- First, close the knob of the gas cylinder and open the vacuum pump knob on the bulb. Evacuate the air filled in the system and by turning on the vacuum pump.
- Once the bulb is emptied, close the vacuum pump knob and switch off the vacuum pump.
- Start filling the bulb with the cylinder gas by opening the gas cylinder knob slowly until the desired difference in the mercury height is achieved. Note the height difference in the manometer. (The value of the height difference should be the same for all the readings.)
- Close all the knobs, also close the connection between the bulb and the manometer to isolate the gas inside the bulb. Disassemble the bulb from the manometer.
- Weigh the bulb on a weighing machine and note the reading down.
- This finishes the procedure for the first gas. Repeat the same procedure for different gases.
Calculation
Calculate the weight of gas (Wg) in the bulb by subtracting the weight of empty bulb (W0) from the weight of the filled bulb (W).
Then calculate the number of moles of the gas as:
The number of moles of all gases should be approximately equal within a small percentage of error. If this is true, then all the gases do obey the Avogadro's law.
If the experiment is performed at STP (T = 273.15 K, P = 101 325 Pa) , then we can also calculate the molar volume Vm as:
And its value should be close to 22.4 dm3 mol−1.
Examples
Example 1
Consider 20 mol of hydrogen gas at temperature 0 °C and pressure 1 atm having the volume of 44.8 dm3. Calculate the volume of 50 mol of nitrogen gas, at the same temperature and pressure?
As from Avogadro's law at constant temperature and pressure,
Therefore, the volume is 112 dm3.
Example 2
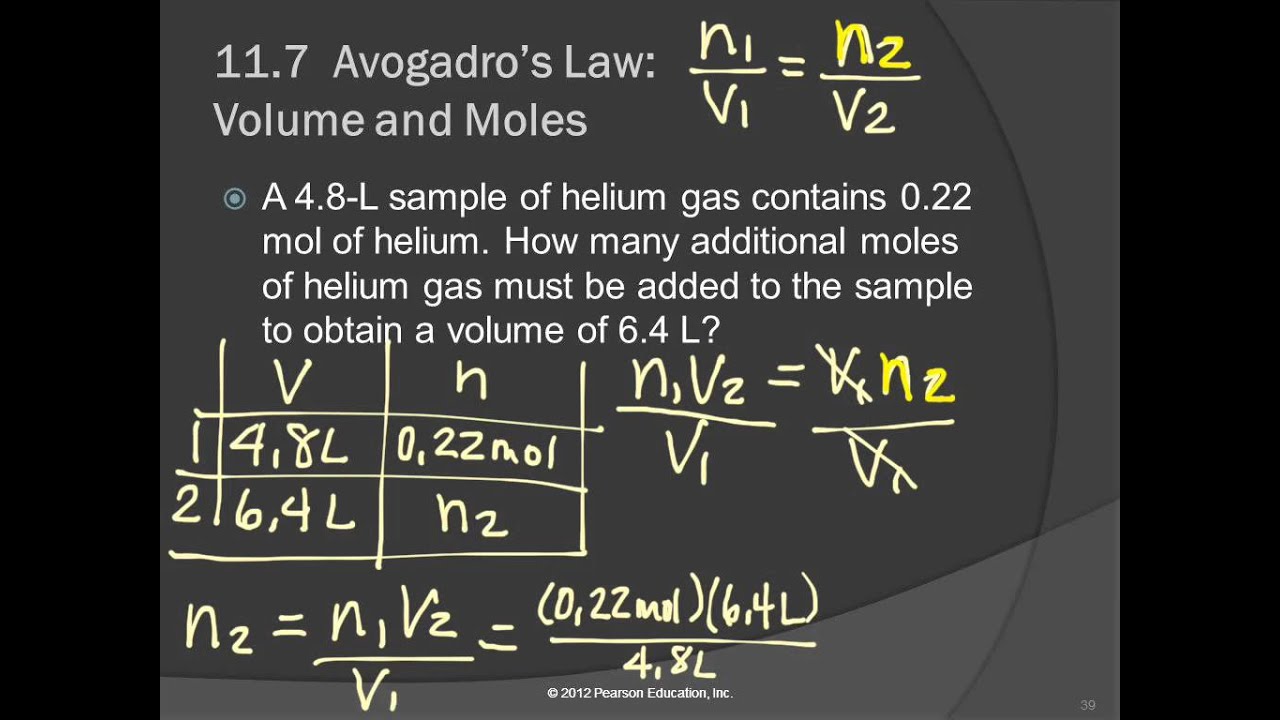
There is the addition of 2.5 L of helium gas in 5.0 L of helium balloon; the balloon expands such that pressure and temperature remain constant. Estimate the final moles of gas if the gas initially possesses 8.0 mol.
The final volume is the addition of the initial volume and the volume added.
From Avogadro's law,
The final number of moles in 7.5 L of the gas is 12 mol.
Example 3
3.0 L of hydrogen reacts with oxygen to produce water vapour. Calculate the volume of oxygen consumed during the reaction (assume Avogadro's law holds)?
For the consumption of every one mole of hydrogen gas, half a mole of oxygen is consumed.
As per Avogadro's law, the volume is directly proportional to moles, so we can rewrite the above equation as:
1.5 L of oxygen is consumed during the reaction.
Associated Articles
Examples Of Avogadro's Law
If you appreciate our work, consider supporting us on ❤️ patreon.- 9
- cite
- response
Copy Article Cite
The field of science has been blooming since the last few hundred years. Revolutionary theories have been proposed, and formulas have been discovered that helped in changing the world for better. I wouldn’t be an exaggeration to say that even small formulas like speed = distance/time have helped in bringing about technological development in the world. One of these revolutionary discoveries is Avogadro’s Law. Examples are the best way to understand anything in science, in this article, we will learn or understand Avogadro’s Law Examples.
Avogadro's Law Formula
Do you know who created Avogadro Law, how and why? No, I tell you, The Italian Scientist Amedeo Avogadro introduced the Avogadro’s Law in 1811.
This law is an experimental gas law. Specifically, this law is for an ideal gas. This law is not applicable to real gases because they show some variations. Understand Avogadro’s Law Examples, Ballons.
Avogadro’s Law
Avogadro’s law states that –
“At the similar and constant physical conditions like Temperature and Pressure, the two different gases like hydrogen and nitrogen in the same volume contain an equal number of molecules.”
How was Avogadro’s Law Influential?
Avogadro’s law was able to explain how equal volumes of gases at ideal conditions gave an equal number of molecules. Although scientists at that time rejected this hypothesis, it gained acceptance after his death, when other scientists realized how important and revolutionary it was in the field of science. You can understand this law with Avogadro’s Law Examples, Lungs, Tyres, etc.
Mathematical Derivation of Avogadro’s Law
From the Ideal Gas Equation,
PV=nRT
Where, P = Pressure, T = Temperature, n = number of molecules of the gas, V = volume of the gas, R= Ideal gas constant (also known as Boltzmann Constant = 0.082057 L atm K-1 mol-1)
Assume P & T = Constant
Aer memories of old soundtrack download. V = n (RT/P)
Where, K = RT/P (Proportionality constant)
V α n
The above equation says that as the volume is increasing, the number of molecules of the gas is also increasing. Or As the volume decreases the number of molecules of the gas is also decreases (like in Ballons). Although Avogadro’s Law can be written in a lot of ways, this relation is the easiest to understand.
At condition 1 (at constant temperature and pressure),
At Condition 1, the Volume of gas is denoted as V1 and the number of molecules of a gas is denoted as n1.
At Condition 2 (P, T = Constant)
At Condition 2, the Volume of gas is denoted as V2, and the number of molecules of a gas is denoted as n2.
Avogadro's Law Equation
According to Avogadro’s law,
V1/n1 = V2/ n2 ————————————————————-(a)
Assume volume V2 is the twice of the volume V1, So,
V2= 2V1 ———————————————————————(b)
Put Value of V2 in equation number (a)
V1/n1 = 2V1 / n2
n2 = 2n1 ——————————————————————–(c) Upgrade movavi video editor 15 plus to movavi video editor plus 2021.
According to equation (c ), If the number of volumes is doubled, then the number of molecules of a gas is also doubled.
Note: This law is for Ideal gases. In real gases, slight variations and deviations occur.
What is Avogadro’s number?
The Scientist Avogadro did not discover Avogadro’s number. The number of molecules in 1 mole is known as Avogadro’s number. Its value in the SI unit is 6.02214129 ×1023 mol-1. And it is denoted by NA. Nowadays, Avogadro’s constants are used instead of Avogadro’s number.
Avogadro's Law Real Life Example
Avogadro’s Graph
Graphical representation of Avogadro’s law,
The above graph is plotted (shows linear relationship) between volume and mole at the constant physical condition. As the volume is increasing, then the molecules are also increasing.
Real-World Avogadro’s Law Examples
Since Avogadro’s law is for ideal gases, people think that it does not have any real-world Avogadro’s Law Examples and that it is just a hypothesis. But that is not the case. Avogadro’s law has numerous real-world applications, and it explains a lot of things that go on in our day to day life.
Lungs
The first real-world Avogadro’s law examples can be seen in our own body. We all know that the lungs help us in breathing. But have we ever wondered about the mechanism? Thanks to Avogadro’s law, it’s easier to understand how our Lungs work.
When we breathe in (inhale), air flows inside our lungs, causing the lungs to expand (increase in volume). From our lungs, the oxygen is extracted and injected into our bloodstream. Then, we breathe out (exhale), causing the Carbon Dioxide (CO2) to pass through, and thus shrinking the volume of the lungs.
Sports
All of us love to play games, right? No? Are you not into sports? It doesn’t matter, but if you’re looking for Avogadro’s law examples, you will find them in games like Football and Basketball. When you blow air into a ball, it causes the volume of the ball to increase, as air molecules start to collect inside of it. And if you puncture the ball, all that air will come out with pressure, causing the ball to shrink and create a loud sound. Avogadro’s law comes into play to explain to us all the things that are going on here.
Tires
Everyone found yourself in a situation where you got stuck in the middle of the road because of a flat tire? Well lucky if you haven’t. Things like puncture, temperature, or time can cause the tire to lose the air inside it, which decreases the volume of the tire and ruins your trip. When you take your car to the gas station, you also get the tires checked. When air is blown into the tires, they get inflated, and the volume is increased, and your car can move smoothly. This is another of Avogadro’s Law Examples.
Balloons
One more Avogadro’s Law Examples is a balloon. Everyone loves balloons. And to bring them to that beautiful state, you have to force air from your mouth into the balloon, causing it to expand to a much higher volume.
Limitations of Avogadro’s Law
Although Avogadro’s law has numerous real-world applications (Avogadro’s Law Examples discussed above) if it also has a lot of limitations. This doesn’t mean that Avogadro’s Law is not accurate; it just means that it is not perfect. So some of the limitations of Avogadro’s Law are listed below,
- The biggest limitation of Avogadro’s Law is that it was introduced to the world, taking into account that it works best for ideal gases. But such a thing as an ideal gas doesn’t exist in the real world. To get perfect and accurate answers, you have to apply this law to ideal gases. For real gases, this law still answers, but the accuracy is often questionable.
- Calculation of Heavy molecules tends to give poor and inaccurate results as compared to lighter gas molecules. This is where the limitation of Avogadro’s law is observed since the calculations for some major heavy molecules turn out to be slightly, or sometimes, mostly inaccurate.
- In real gases, temperature plays a significant role in increasing the ratio of volume to the mole as compared to ideal gases. This is because, in real gases, a temperature increase causes the gas to expand because of the intermolecular repulsion forces.
Avogadro’s law helps chemists and physicists in finding more theories and exploring new areas of chemistry.
